


Figure 1. The riders in the Rainbow amusement ride move in a circle around a horizontal axis.
Invited chapter, in Science Education in the 21st Century ( Nova Science Publishers, NY, Ed. Ingrid V. Eriksson)
Newton's discovery of the laws of motion changed the way we view our universe. They are a standard part of any physicist's toolbox. Still, there is an overwhelming body of evidence that students often fail to master even the most basic aspects and everyday experience often appears to contradict the laws of motion.This chapter focuses on acceleration, which, unlike velocity, can be detected by a body in motion and measured within the moving system. Acceleration is also more fundamental; it is absolute, whereas velocity is relative and depends on the frame of reference chosen. This makes it possible to build on, rather than reject, the experience by learners and start in situations which where everyday observations do not contradict Newtonian concepts. The vector character of acceleration does not need to be seen as an abstract concept, but is evident in our daily three-dimensional motions, without the need to invoke a formal mathematical treatment.
After an introduction presenting different aspects of acceleration, including different ways of measuring and experiencing acceleration in different types of motion, follows a presentation of an investigation of new physics students' understanding of acceleration. Consequences of introducing acceleration through the mathematical definition, a=dv/dt, or through Newton's second law, a=F/m are discussed and related textbook presentations are analysed. Which aspects of acceleration are discerned and which are not? Can teaching be modified to make critical aspects of acceleration accessible to the students?
The last part of this chapter describes pilot projects with 10-11 year olds who were invited to guided physics experiments in an amusement park or playground. Is it possible and meaningful for them to investigate acceleration in three dimensions?
Newton's discovery of the laws of motion changed the way we view our universe. The laws are a standard part of any physicist's toolbox. They are also among the most studied part of physics education and there is an overwhelming body of evidence that students often fail to master even the most basic aspects (see e.g. McDermott, 1998, McDermott and Redish, 1999, Redish, 2003). Can anything new be added to the research on learning and teaching mechanics? What if there is something fundamentally wrong in the approach to force and acceleration in school physics?
The laws of motion are traditionally introduced through non-motion, discussing forces on a system at rest. The study of forces then moves on to situations where all forces cancel. If the body is not at rest, it thus remains in uniform rectilinear motion and Newton's first law seems to contradict everyday experience, where a cyclist needs to pedal to keep going, and a car comes to rest unless the engine continues to provide energy.
Would it be possible to approach the teaching of the laws of motion by focusing instead on acceleration, related to force through Newton's second law? From a physics point of view, acceleration is more fundamental than velocity; it is absolute, whereas velocity is relative and depends on the frame of reference chosen. Acceleration, unlike velocity, can measured within the moving system. It can be detected by a body in motion and the "body" can be that of a learner. This makes it possible to build on, rather than reject, the experience by learners and start in situations which where everyday observations do not contradict Newtonian concepts. The vector character of acceleration does not need to be seen as an abstract concept, but is evident in our daily three-dimensional motions, without the need to invoke a formal mathematical treatment.
The investigations in this work are inspired by the theory of variation (Marton & Booth, 1996, 1997). The theory of variation builds on the phenomenographic tradition, where the researcher explores and cathegorizes different ways of experiencing a phenomenon. In the continuing development of phenomenography, the need for the learner to experience qualitative variation is emphasized as a key to learning and awareness. The variation of important aspects of the phenomenon of interest is essential for the learner to be able to develop meaning and a structure to observations. More recently work in that field has focused on what essential variation around critical aspects was offered to the students in a teaching situation: what constitutes the space of learning (e.g. Runesson, 2006, Ling et al, 2006).
The present work is restricted to analysis of the variation in students' understanding in connection to the aspects available from textbook presentations. Possible alternative strategies for teaching motion to younger learners are suggested, which would allow additional variation. Lessons have been implemented in a few pilot cases, but not yet evaluated systematically on a larger scale.
Section 2 gives a backgrund to the student group in focus, in terms of results on the common "Force Concept Inventory". A description of the use of amusement park activities for physics learning is followed by an summary of important aspects of acceleration and measurements. Section 3 presents student replies, collected through a quiz in class, to a questions concerning a relatively simple situation, with uniform circular motion in a vertical plane, used as a starting point for the investigation of their understanding. Group interviews are used to study and challenge the views of acceleration, starting from an open question. Interview excerpts are presented and discussed in Section 4. The student difficulties observed are then analysed in terms of the variation (or lack of it) which is available to the students from textbook presentations, as discussed in Section 5. Possibilities to expose younger learners to a wider variation of aspects of force and acceleration are explored in Section 6, including suggested experiments and interviews with 10-year olds who have tried them.
The main questions in this work are
The engineering physics students regularly obtain an FCI score around 80 % as they start their education at Chalmers. This seems to be higher than any pre-test result in the study by Hake (1998). For other programmes where we have administered the test, including the university physics course at GU, the chemistry with physics and the chemistry/biology engineering programmes at Chalmers, the FCI scores have been much lower, around 55% at entrance.
In spite of the high entrance scores for engineering physics students, exam results have not matched expectations. A comparison between individual results indicates that a good FCI scores is a necessary, but not sufficient, condition for good exam results (Pendrill, 2005). During exam grading, it was obvious that mathematics skills also play an important role. The importance of mathematics also for conceptual understanding was emphasized further in an interview study (Adawi et al 2005). These results have been a strong incentive to analyse students' conceptual problems in more detail, and to invent ways to help students develop a deeper understanding of fundamental concepts.
Experiments have also been designed for younger learners, with the intention to use the controlled motion in an amusement ride to illustrate fundamental physical principles, as discussed in Section 5, which also presents excerpts of group interviews after a visit. Section 5 also discusses observations from a physics lesson in a playground. More details about the amusement park project can be found in previous work (Bagge & Pendrill 2002, 2004, Bagge, 2003, Nilsson et al. 2004, Pendrill, 2005, Pendrill and Rödjegård, 2005) and at the project WWW-site (Pendrill, 2007).
The experiences from real life are rarely from frictionless surfaces or without air resistance; the bicycle comes to a stop unless you pedal, the puck stops sliding even on very smooth ice and a feather falls to the ground slower than the stone. The Aristotelian world view of a force needed to maintain a constant velocity, and bodies falling with velocity depending on their mass, is a natural interpretation of years of continuous observations. It is given many years to develop before the physics teacher tries to interfere. Yet, acceleration, in its full vector capacity, is very much a part of everyday life. The child in the swing, on a slide or see-saw, or jumping down a from a climbing rack enjoys the sensation of changed acceleration and interplay of forces - without feeling the need to refer to Newton's laws.
Accelerometers can help develop insights for the understanding of force and acceleration. Acceleration as the time derivative of velocity can be obtained from a measurement of velocity or distance relative to another object, e.g using an ultrasound ranger. Such a measurement gives the difference in acceleration between the two systems. However, accelerometers measure acceleration without the need for an external reference, by building on Newton's second law in the form a=F/m. Simple mechanical devices can give a direct real-time visual measurement, reflecting the "g-force" experienced by the rider. For purely horizontal motion, acceleration can be obtained by measuring the angle to the vertical for a free-hanging pendulum - for young learners, this can be a soft toy on a string. Vertical acceleration can be measured through the extension of a spring. For illustration and qualitative measurement, this can be a small plastic "slinky".
Electronic accelerometers have the advantage of giving a data set that can be analysed in the classroom. They may use the changed distance between two condensator plates to obtain a voltage readout which is translated to acceleration. Acceleration in three dimensions can be obtained by combining three such devices Accelerometers, in spite of their name, do not measure pure acceleration, but rather components of the vector a - g, which can be seen as the non-gravitational force per unit mass. The values for the different components obviously depends on the orientation of the probe. A complete description of motion for a "motion tracker" or "inertial navigation system" requires also a way to measure rotation around the axes.
Which aspects of acceleration made are available to physics students in school teaching? What are the critical aspects and can a changed teaching strategy help students discern them?



Figure 1. The riders in the Rainbow amusement ride move in a circle around a horizontal axis.
For motion in a circle with constant angular velocity, the acceleration is always directed to the center and the resultant force, obtained by combining the force from the ride on the rider with the gravitational force, mg, should then always be directed toward the center. The magnitude of the centripetal acceleration does not change. In order for the rider to experience weightlessness at the top, the centripetal force must have a magnitude mg.
This task has been found to expose an incomplete understanding of forces. One aspect that is found to be problematic is that the force from the ride is neither purely horizontal or purely vertical (except at the top and bottom of the circular ride). On the sides, the total force from the ride is pointing 45o above the horizontal, towards the top of the circle.
Table 1 shows the results for three cohorts of engineering physics students. (In 2004 and 2005, the test was handed out only in subgroups of students.) A number of typical diagrams could be identified. The distributions of different types of replies is shown in Table 1. Even students mastering the apparent weightlessness at the top and the 2mg normal force at the bottom often fail to include all forces in the points to the left or right or in an arbitrary point. A number of students have drawn two additional forces at the sides, one upward force to counteract gravity and one inward force to provide the centripetal acceleration. These replies have been classifed as correct, but counted separately ("pair of forces"). Some students consistently assigned a normal force exactly cancelling the force of gravity. Other students included only a centripetal force of the same magnitude as the force of gravity. Not surprisingly, a centrifugal force was used in a few replies - but only in a total of two cases it was used in a consistent way. The Aristotelian concept of a force in the direction of motion ("velocity force") occurs in a few cases.
| Table 1: Percentage of students drawing different types for force diagrams as discussed in more detail in the text. (Only students answering the question have been counted) . In 2004 and 2005, the test was administered just before the amusement park project presentations, whereas in 2006, it was given before the students had written their project reports. The amusement park project in 2004 was only loosely connected with the course. | |||||||
| Group | Correcta (pair of forces) | |N| =m |g| | m g and Fc | Centrifugalb | Correct, vertical | "Velocity force" | Misc. |
|---|---|---|---|---|---|---|---|
| F students (2004) (N=68) | 19(6) | 10 | 38 | 7(1) | 6 | 7 | 12 |
| F students (2005) (N=32) | 36(15) | 15 | 33 | 3 | 12 | 0 | 0 |
| F students (2006) (N=89) | 36(23) | 0 | 26 | 7(1) | 15 | 9 | 8 |
The force diagrams drawn for the Rainbow ride show that students do not automatically connect the experience of the body to the forces in Newton's second law. It also shows that many students treat the centripetal force as an interaction on its own, rather than as a resultant to real forces. In spite of high entrance FCI-scores, only about one third of the students drew consistent force diagrams in this relatively simple situation.
Comparison between the results for the different student groups show a significantly lower fraction of correct results for the F(2004) students. For this student group, the amusement park project was more loosely connected with the rest of the course. The importance of integration of informal learning activities with other course work, through preparation and follow-up, is known from other cases of informal learning (See e.g. Rennie and McClafferty, 1996). Discussion and follow-up, including challenges to students' descriptions, are important also to help students focus on important features of force and acceleration in connection with the rides.
Just preceding this discussion, the students had been discussing forces in a swing, and show the force diagram as the teacher enters.
An important dichotomy is whether the description of acceleration refers to another object or involves the own body. In these interview excerpts, only one reference is made to the experience of the body. Is the human body an underutilized teaching tool in mechanics? The next section investigates to what extent are textbooks make use of it.
One-dimensional vertical motion is usually restricted to free fall starting from rest. The accelerating objects are mainly balls, stones and unspecified objects, possibly a planet or a runaway elevator. Two-dimensional motion is usually restriced to projectile motion, uniform circular motion and possibly a pendulum.
In engineering textbooks on mechanics, an extensive treatment of statics often precedes the study of motion. In the "dynamics part", Newton's second law could be perceived as a giving correction terms to Newton's first law, where the presence of acceleration is dealt with by including inertial forces in the accelerated systems.
University physics textbooks are more likely to focus the Copernican revolution, with motion of planets playing an important role in the "clockwork universe". Galilean invariance is given a more prominent role and Newton's first law, with absence of forces, is seen as a special case. Independent of approach, the "body" is rarely human.
Below, we consider in more detail the way undergraduate textbooks deals with the aspects of motion that can be measured from within moving systems, i.e. acceleration and rotation, and that are needed for a complete "motion tracking".
Provided that the observations take place in a small region of space and time, it is not possile by experiment to distinguish between an accelerating frame and an intertial frame in a suitably chosen gravitational potential.Halliday et al (1997, p205) quotes Einstein's statement "I was in the patent office at Bern when all of a sudden a thought occurred to me: 'If a person falls freely, he will not feel his own weight. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation'."
In the older, less colorful but more mathematical, treatment in Alonso and Finn (1992), the equivalence principle is introduced already in connection with the treatment of gravitation, with reference to Galileo.
Following the lead provided by Einsteins own textbook (1916), the most common example after the introduction of the equivalence principle is to consider elevators; elevators on the ground, free-falling elevators and elevators accelerating in deep space. Free-falling astronauts in orbits are also common.
Textbooks sometimes bring up the possibility to simulate gravity in a rotating space station. Hewitt (2005) is relatively unusual in including a discussion about the inhomogeneity in the apparent gravitational field if the space station is too small (p160). He discusses the fictive "centrifugal force" (p147) and emphasizing that it is as real as gravity to the person in a rotating frame, spending about 3 pages. Fishbane et al (2005) very briefly mentions centrifugal force, in less than half a page (p141). In most textbooks considered, the term "centrifugal force" is avoided. Of the engineering dynamics books studied (Meriam and Craige, 2003, Hibbeler, 2004, Bedford and Fowler, 2005) only Meriam and Craige (2003) mention the centrifugal force, and none introduces the equivalence principle.
How do textbooks treat "g-forces"? The only explicit example found is Halliday et al (1997, p66) who introduces "g-induced loss of consciousness", discussing the exposure of fighter pilots in loops and other maneuvers. They also refer to the experiment where Colonel John P Stapp was rapidly accelerated in a rocket sled and then braked to stop, being exposed to many g. The text emphasizes that it is not the speed causing discomfort. They also mention that acceleration is sometimes expressed in terms of "g units" (p17). In a later edition, Colonel Stapp is still included, but the fighter pilot example has been removed.
Although the fictive centrfugal force is mentioned in many of the books, the accompanying fictive force for moving bodies, the "Coriolis force" most often evades treatment in the college books. Searching the index for "Coriolis" brings one end-of-chapter problem in Fishbane et al. (2005). Giancoli (2005) gives an appendix discussing fictive forces, including a discussion of a ball rolling on the surface of a carousel. Serway and Beichner (2000) includes, in the context of pendulum motion, a photo of a science center Foucault pendulum, with a brief description of the history. Alonso and Finn (1992) spends about 3 pages on the Coriolis effect, including the the formula, a discussion of the Foucault pendulum and reference to weather systems.
All "Engineering Mechanics: Dynamics" books studied discuss the Coriolis effect in connection with moving machine parts, which is also what led Coriolis to consider it. Meriam and Craige (2003) includes an end-of-chapter problem involving the effect on a train moving north. Bedford and Fowler (2005) spend three pages discussing the effects in different parts of the world and the consequences for weather systems. None of them mentions the Foucault pendulum.
It can also be noted that commonly used tests, including the Force Concept Inventory discussed in 2.1, include no reference to the experience of the body.
In universities, mechanics is sometimes treated as part of mathematics, and mathematics is, indeed, an extremely useful tool to describe and find motions and forces. Mechanics may also be classified as "mathematical physics", and this tradition seems to penetrate school book presentations. An concern is that a mathematical approach may hide the connection to physical reality - which systems accelerates or rotates for relative motion? In addition, the purely mathematical approach clearly is not suitable for young learners. Young learners know of "g-forces", which connect directly to the experience of the body. However, they are very unlikely to be able to read about g-forces in a physics textbook.
The common initial focus on one-dimensional motion, which is a natural consequence of the mathematical approach, obscures important aspects of the concepts. Acceleration as a change of direction is not an obvious possibility in a simplified treatment where motion is studied only in one dimension. However, already in one dimension, failure to distinguish between speed and velocity leads to confusion: Many are the teachers who have been frustrated by the large number of students who state that the acceleration is zero in the highest point for a ball thrown up in the air - and are sometimes relucant to give up their view. Indeed, if retardation is described as different from acceleration, neither applies to the situation where the body is at rest. Defining retardation as "negative acceleration" implies a definition of acceleration as derivative of speed, i.e. of |v|. In classroom discussions it is obvious that this concept is very well rooted among students, who also often incorrectly insist that the acceleration as a swing pass the lowest point must be zero, since the speed has a maximum, and forget all about the centripetal acceleration.
One undergraduate textbook states that "accelerometers" can be used as a navigation device because the measurement of acceleration can be turned into a measurement of position”. This statement is true only in one dimension. In three dimensions, also rotation around three axes must be considered to obtain a "motion tracker" (as described e.g. by Rödjegård and Pendrill, 2005). Nevertheless, in discussions with students and colleagues, I often encounter the view that a three-dimensional accelerometer measurement would give a complete description of the motion, e.g. in a roller coaster. In an acceleration-based mechanics education, the focus on what can can be measured is natural, and opens for comparison with familiar situations.
Foucault's classical pendulum experiment in Pantheon in 1851, demonstrating the rotation of the earth, risks to goes unnoticed in most first-year physics textbooks. The replies of new students to the question "How do we know that the earth rotates around its axis?" are consistent: Most students happily give a reply involving day and night, not reflecting that day and night on earth are much older than the insight of a spinning earth. The omission of Foucault's pendulum can thus be seen as a missed opportunity to illustrate physics as an experimental science, and to train students to reflect on "How do we know?".

| Figure 2. Miniature "Foucault" pendulum experiment in a playground carousel. |
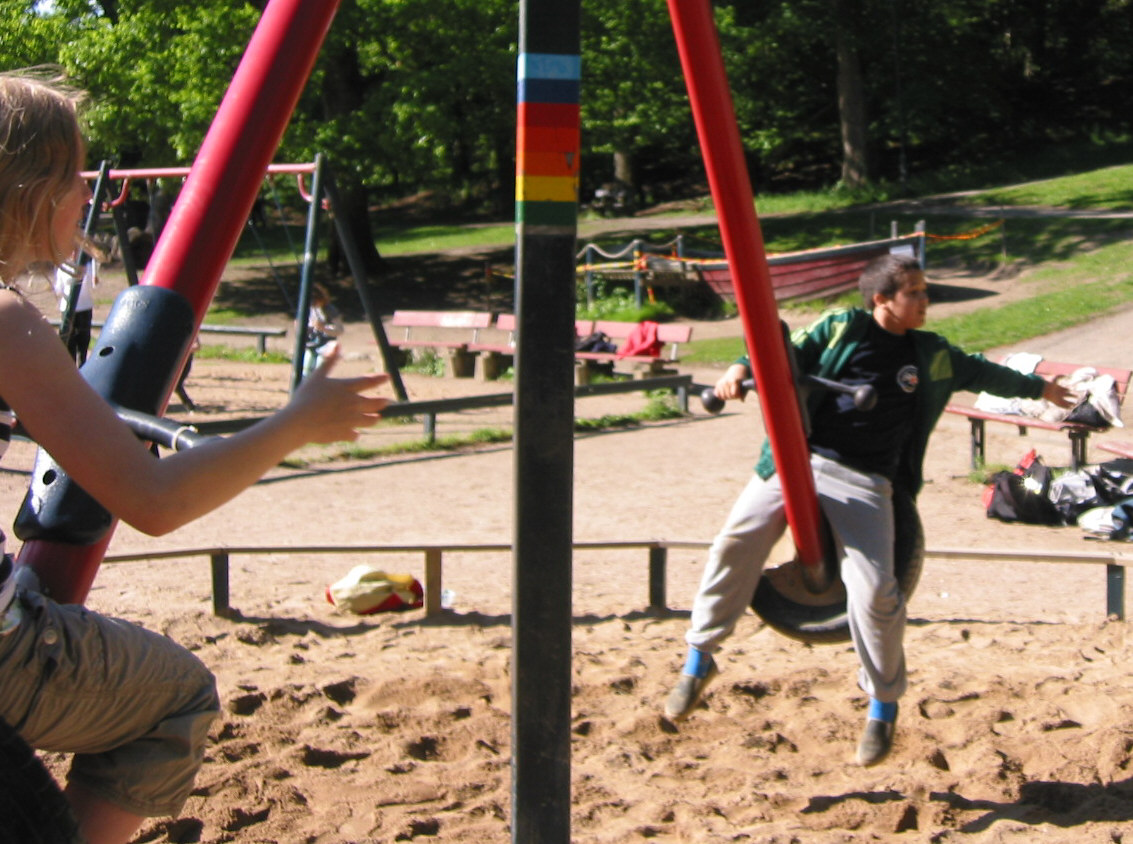
| Figure 3. Two fourth graders throwing ball in a playground carousel which rotates counter-clock-wise. In the first attempts, the ball arrives behind the person on the other side. |
In planning lessons on acceleration for young learners, it is natural to build on strongly visual experiments and, if possible, also on the experience of the body. What can be learned by fourth-graders (10-11-year olds) from a lesson in a playground or in an amusement park? Earlier group interviews in connection with such activities have been analysed e.g. by Bagge and Pendrill (2002), Nilsson et al (2004), and Nilsson (2005).
A playground has the advantage that it can be visited frequently and experiments can be repeated if additional questions arise during follow-up discussions after the visit. Recently, a class of forth-graders were invited to experiment at a large playground in Göteborg. Experiments were also performed in swings and slides (Pendrill and Williams, 2005) and in the climbing rack. At the end of the visit, the whole class sat down and discussed the experience, and were asked what experiments had surprised them most.
In 2001 a class of 10-year olds were invited to experiment at Liseberg. All children were well familiar with the park from previous visits with their families. They had prepared the visit by writing letters with questions to a ride of their choice. This approach was suggested by the teacher, as a way to enable teaching to be more closely based on childrens thoughts. Below, we discuss their experiences in connection with two rides with vertical acceleration.
At the end of the playground visit, which included also forces in swings and bottle races down a slide (Pendrill and Williams, 2005), the class gathered and was asked what experiment they had found most surprising. The carousel was a clear choice, and they could discuss how they had to change the way to throw the ball in order to reach the person on the other side.

| Figure 4. Visual g-force measurements in the Frog Hopper, discussed in 6.2. |
For the Circus Express roller coaster we meet again children who associate long slinkys with high speed, and other children who are more precise in their observations, e.g.:
The first quotes quotes for both rides illustrate the common need for a dialogue to find out what children mean by "went down" and "went up" in their descriptions. "Went down" can sometimes be used to describe the situation when the ride turns to go down, i.e. at the top. The comment about "so much speed" may indicate an Aristotelian expectation of a force needed to maintain speed, but could also mean that the ride had "so much speed" that needed to change. In the recorded interviews, these points were not pushed by the interviewer.
If possible, a dialogue to help children give more precise descriptions should take place directly after the ride, when memories are fresh and where children still have a chance to repeat the experiment. In the park, they can also observe the variation of the length of a slinky held by a friend in the Frog Hopper, making possible a joint discussion on the ground for the rest of the group.
The carousel experiments described above give first-hand experience with the fictive "Coriolis force" acting on objects moving in a rotating coordinate frame. The Coriolis force is certainly not part of the school curriculum. In college textbooks, it is in general not mentioned, except possibly in one of the end-of- chapter problems. When students encounter the mathematical treatment at university, in general, they have no direct experience of it, and find it a very abstract phenomenon, which little relevance for life nor technology. Still, experiments in slowly rotating system have been found to fascinate 10-year old learners (as well as older students), even if it did not relate directly to any question in their initial letters. The interest was created by the learning situation prepared in the park.
The carousel was also the experiment which made the strongest impression for the class doing the playground visit. They were absorbed by watching the little pendulum swing. They had also figured out that they needed to throw the ball a little bit to the front of the friend on the other side, since he would have moved a bit before the ball arrived.
The two amusement rides with vertical acceleration discussed above involve motion in one and three dimensions. In these rides children had the possibilities to measure g-forces for themselves. The replies are relatively similar for both rides, and also consistent with the experience from discussing with classes in the park, at these rides and also e.g. in the classical Rainbow ride discussed in Section 3. We see no evidence that the added spatial dimensions of the ride complicate observations. In fact, the accompanying horizontal movement makes it possible to use a small drawing of track of the roller coaster to ask children to be more precise about the points where they feel heaviest or lightest.
The use of a real time visual measurement makes it easier to discuss the experiences with a group of learners. This is particularly useful where observers can view the whole ride, or at least a significant part of it. In this way, the experience benefits also children (and sometimes teachers) who are reluctant to go on the rides. Children seem to have no difficulty associating their apparent weight with the length of the slinky, and thus make an initial connection between force and acceleration.
A dialogue is important for sharpening observations and conclusions and to clarify distinctions between different parts of the motion. This is no argument against 10-year olds performing the experiments. Replies similar to those quoted here are given by physics learners throughout school.
New students' description of acceleration, as it shows in group interviews, indicates in most cases a very limited relation to the concept, which is unlikely to enable students to use it to understand new situations.
Students often forget the vector character of velocity and acceleration. Acceleration as a change of direction of the velocity is not an obvious possibility in a simplified treatment where motion is studied only in one dimension. However, already in one dimension, failure to distinguish between speed and velocity leads to confusion: Many are the teachers who have been struck by the large number of students who state that the acceleration is zero in the highest point for a ball thrown up in the air. Indeed, if retardation is described as different from acceleration, neither applies to the situation where v=0 and the concepts can not be generalised. If acceleration were derivative of speed, i.e. of |v(t)|, then retardation would imply negative acceleration and the derivative would be undefined for v=0.
Thus, the restriction of discussions of acceleration to one-dimensional motion and to situations where the body starts from rest, not only excludes most everyday experience of acceleration, but may also lead to views that get in the way of an understanding of the more general concept. Although a mathematical description of 2 or 3 dimensional motion should not be attempted before the 1-dimensional situations are understood, there are alternative ways of viewing and introducing acceleration that makes the concept accessible also to younger learners.
The formulation of Newtons second law as a=F/m shifts the focus from force to acceleration. Whereas the forces acting on mother and child e.g. in an amusement park ride are very different, they may both share the same acceleration. They also experience the same "g-force".
The common school focus on one-dimensional motion alienates school physics from everyday life experiences. This focus is also found to obscure important properties and to encourage ways of thinking that can not be applied to more general cases. Some misunderstandings that may be traced to the textbook treatments have been found to be very persistent. Focusing on acceleration, rather than on uniform, rectilinear motion makes it possible to build on the experiences of the body. Simple experiments are presented, which have been tried out in pilot projects in a few classes. These experiments relate to important physical principles and introduces a distinction between relative and absolute motion.
Experiments during a couple of hours in a park or on a playground can only be first steps toward an understanding of acceleration. Focusing on acceleration, rather than on uniform, rectilinear motion, makes it possible to build on the experiences of the body, offering qualitatively different ways of understanding the concept of acceleration. Observations and interviews show that physics experiments in a playground or amusement park can be both enjoyable and educational also for young learners. We hope that inviting children to make a distinction between acceleration, velocity and speed at an early age, can help them to interprete future observations in a more Newtonian way. The analysis in this work shows that the experience of the body is a severely underused resource for teaching about force and motion.